I am excited to announce the Upper School Mathematics Students of the Trimester – Winter 2021!
Each math faculty member was free to choose whichever student of theirs they thought best exemplified what they are looking for in a model mathematics student. The official description of the award is as follows:
“Awarded to students who exemplify the math department’s core values of competence, confidence, and perseverance while helping their peers realize the relevance and importance of an exceptional mathematical education both for its beauty and for its practical application.”
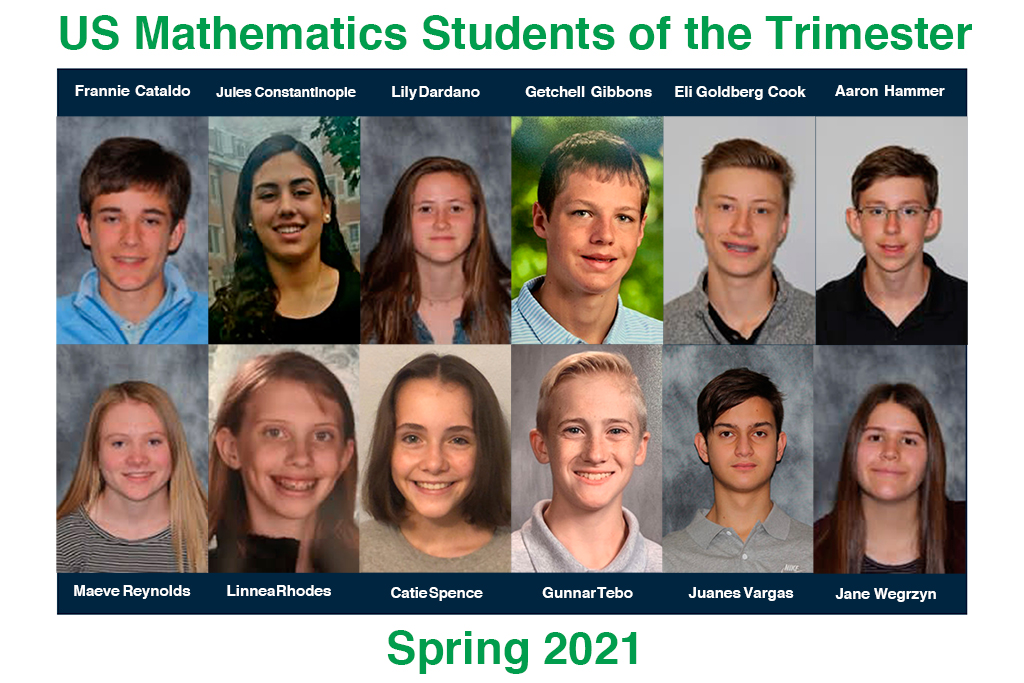
The following students have been recognized as the Upper School Mathematics Students of the Trimester for Spring 2021.
Please join me in congratulating these outstanding mathematics students!
 Frannie Cataldo – Frannie has been a very strong student all year long. He learns material quickly and is able to apply his knowledge well. He is also very humble yet is always willing to lend his insights into the material with the rest of his classmates. Frannie Cataldo – Frannie has been a very strong student all year long. He learns material quickly and is able to apply his knowledge well. He is also very humble yet is always willing to lend his insights into the material with the rest of his classmates. |
 Jules Constantinople – Jules had a fantastic third trimester in Algebra II. She possesses a strong work ethic and is very engaged during class. I am particularly impressed by her ability to stay resilient. When Jules encounters a challenge, she figures out how to overcome it. Jules wants to get better each day and is willing to put in the work necessary to do this, which will serve her well in future math classes. Jules Constantinople – Jules had a fantastic third trimester in Algebra II. She possesses a strong work ethic and is very engaged during class. I am particularly impressed by her ability to stay resilient. When Jules encounters a challenge, she figures out how to overcome it. Jules wants to get better each day and is willing to put in the work necessary to do this, which will serve her well in future math classes. |
 Lily Dardano – Lily challenged herself all year to produce the highest quality work on each assignment. She was the main contributor to class in terms of individual and group contributions. Her level of understanding of the material was one of the highest in the class because of her desire to truly understand the material. Lily Dardano – Lily challenged herself all year to produce the highest quality work on each assignment. She was the main contributor to class in terms of individual and group contributions. Her level of understanding of the material was one of the highest in the class because of her desire to truly understand the material. |
 Getchell Gibbons – Getchell approached this class, and programming in general, with the intention to extend his understanding of the fundamental programming constructs by pushing himself in each area. His solutions to problems incorporated not just past material but ideas that he explored well beyond the minimum required. Getchell Gibbons – Getchell approached this class, and programming in general, with the intention to extend his understanding of the fundamental programming constructs by pushing himself in each area. His solutions to problems incorporated not just past material but ideas that he explored well beyond the minimum required. |
 Eli Goldberg Cook – Congratulations, Eli, on this well-deserved honor. You have just completed an amazing year in Algebra II and more specifically, a spectacular third trimester. Always engaged in class, you have provided your peers with exceptional leadership and demonstrated how to be fully engaged each day in class. You have developed strong algebra skills and fostered a genuine curiosity for math. The incredible results from your evaluations showed that you could transfer your knowledge accurately on a range of topics as you strived to be your very best. It was a joy to watch you develop as a math student and I appreciate your eagerness to challenge yourself. Confident but never arrogant, you made our class better each day with your thoughtful participation. Well done! Eli Goldberg Cook – Congratulations, Eli, on this well-deserved honor. You have just completed an amazing year in Algebra II and more specifically, a spectacular third trimester. Always engaged in class, you have provided your peers with exceptional leadership and demonstrated how to be fully engaged each day in class. You have developed strong algebra skills and fostered a genuine curiosity for math. The incredible results from your evaluations showed that you could transfer your knowledge accurately on a range of topics as you strived to be your very best. It was a joy to watch you develop as a math student and I appreciate your eagerness to challenge yourself. Confident but never arrogant, you made our class better each day with your thoughtful participation. Well done! |
 Aaron Hammer – Aaron is the undisputed class leader for Honors Geometry. The group looks to him for help and he is always ready to assist with a problem. He shows good work on his problems and has steadily improved his ability to explain work to others. Aaron is a great example of a fully involved discussion-based-learning student. Aaron Hammer – Aaron is the undisputed class leader for Honors Geometry. The group looks to him for help and he is always ready to assist with a problem. He shows good work on his problems and has steadily improved his ability to explain work to others. Aaron is a great example of a fully involved discussion-based-learning student. |
 Maeve Reynolds – Maeve’s work improved throughout the year. This trimester she really pushed herself to work outside her comfort zone, asking questions and contributing to the class conversation more than before. Her work was exemplary because of her collaboration with classmates enhancing her own understanding. Maeve Reynolds – Maeve’s work improved throughout the year. This trimester she really pushed herself to work outside her comfort zone, asking questions and contributing to the class conversation more than before. Her work was exemplary because of her collaboration with classmates enhancing her own understanding. |
 Linnea Rhodes – All year, Linnea has dug into the material. She was always willing to dig even more when she found a concept that challenged her. During T3, she made it a point to really work on organizing her work as we used multiple strategies and formulas in one problem. She’s a creative thinker, willing to offer up solutions (even if she is unsure about it) and ready to adapt her thinking. Linnea Rhodes – All year, Linnea has dug into the material. She was always willing to dig even more when she found a concept that challenged her. During T3, she made it a point to really work on organizing her work as we used multiple strategies and formulas in one problem. She’s a creative thinker, willing to offer up solutions (even if she is unsure about it) and ready to adapt her thinking. |
 Catie Spence – Catie has always been a strong student in Algebra 2 Honors, but this spring, she aced pretty much every assignment the class had. While she’s quiet in class, she is always ready with an answer when she’s called on, and then her answer is almost always correct! She’s been a delightful addition to the class – a gifted mathematician who supplements her ability with diligent effort and attention to detail. Great work this spring! Catie Spence – Catie has always been a strong student in Algebra 2 Honors, but this spring, she aced pretty much every assignment the class had. While she’s quiet in class, she is always ready with an answer when she’s called on, and then her answer is almost always correct! She’s been a delightful addition to the class – a gifted mathematician who supplements her ability with diligent effort and attention to detail. Great work this spring! |
 Gunnar Tebo – Gunnar joined App Programming with no experience coding, but you would never have known! He exemplified all of the skills this class aims to teach: coding, creativity, resourcefulness, and determination. Gunnar’s final project game also showed his ambition and eagerness to extend his learning beyond what we covered in class. Gunnar Tebo – Gunnar joined App Programming with no experience coding, but you would never have known! He exemplified all of the skills this class aims to teach: coding, creativity, resourcefulness, and determination. Gunnar’s final project game also showed his ambition and eagerness to extend his learning beyond what we covered in class. |
 Juanes Vargas – Juanes is a very strong math student, but what makes him shine is his deeply embedded determination to completely consume every math topic he encounters. Juanes is not satiated by just knowing how to express the answer to a question. Instead, he only rests when he reaches a point when he can teach someone else how to do the problem, all while expressing where the logic of the topic comes from and how it can be creatively applied. Juanes does not rest on his Calculus abilities. He is always pushing himself to go further in a healthy, productive, and inspiring way. Juanes Vargas – Juanes is a very strong math student, but what makes him shine is his deeply embedded determination to completely consume every math topic he encounters. Juanes is not satiated by just knowing how to express the answer to a question. Instead, he only rests when he reaches a point when he can teach someone else how to do the problem, all while expressing where the logic of the topic comes from and how it can be creatively applied. Juanes does not rest on his Calculus abilities. He is always pushing himself to go further in a healthy, productive, and inspiring way. |
 Jane Wegrzyn – Jane had arguably the best detail in her work out of any of my AP Statistics student this year. This detail translated to Jane consistently scoring at the top of my class on assessments. For these reasons and more, I am proud to make Jane my math student of the trimester! Jane Wegrzyn – Jane had arguably the best detail in her work out of any of my AP Statistics student this year. This detail translated to Jane consistently scoring at the top of my class on assessments. For these reasons and more, I am proud to make Jane my math student of the trimester! |
Past students of the trimester can be found right here: Fall 2013, Winter 2014, Spring 2014, Fall 2014, Winter 2015, Spring 2015, Fall 2015, Winter 2016, Spring 2016, Fall 2016, Winter 2017, Spring 2017, Fall 2017, Winter 2018, Spring 2018, Fall 2018, Winter 2019, Spring 2019, Fall 2019, Winter 2020, Spring 2020, Fall 2020, Winter 2021
 A few days after each AP Calculus BC exam, the College Board releases the free response questions from the exam. They don’t release their very succinct answer keys for a few more weeks… so… I had my students make their own answer keys as well as screen recordings of their solutions!
A few days after each AP Calculus BC exam, the College Board releases the free response questions from the exam. They don’t release their very succinct answer keys for a few more weeks… so… I had my students make their own answer keys as well as screen recordings of their solutions!














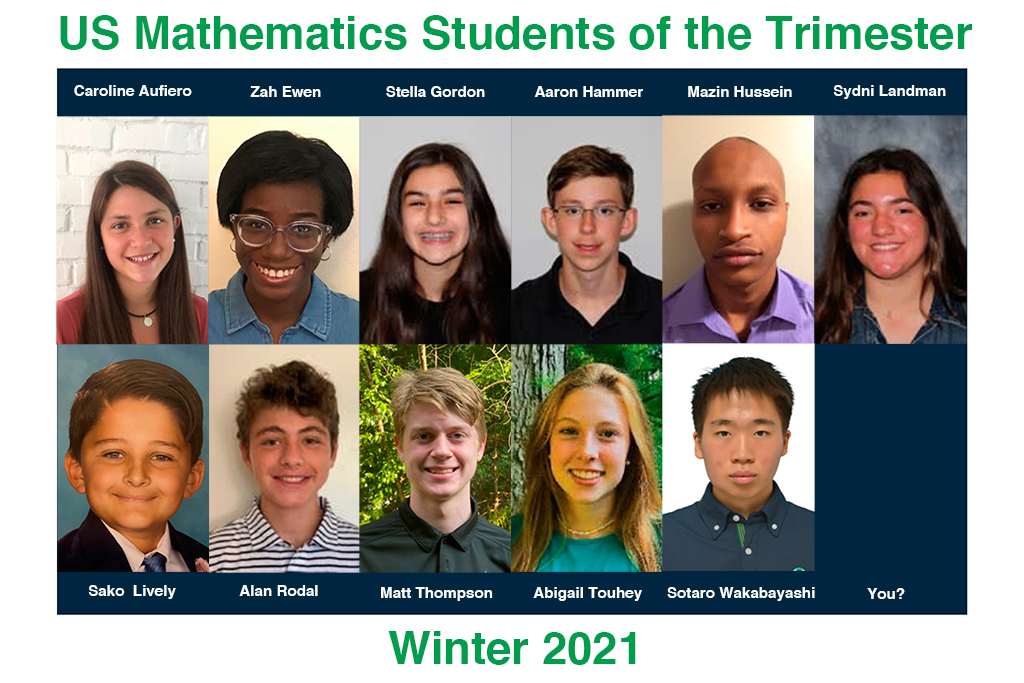













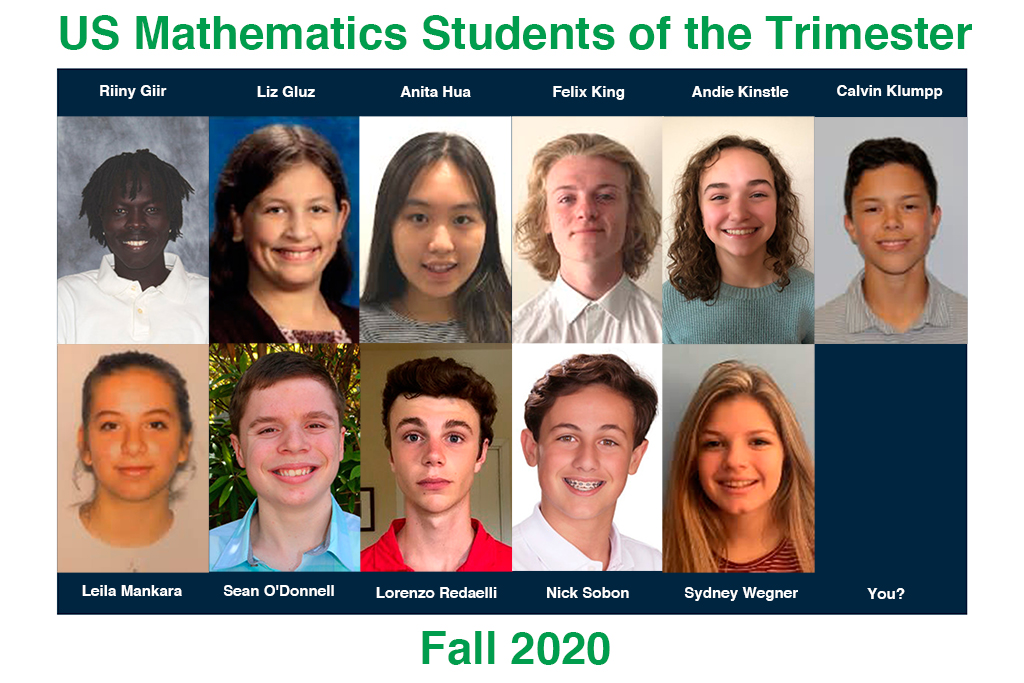
 Riiny Giir – Riiny excelled in both classes. He has an exceptionally clear understanding of coding and problem solving. In the Robotics class, he chose to work with MicroPython in the Mindstorms setting. This was taking a definite risk and will prompt a curricular change to that language!
Riiny Giir – Riiny excelled in both classes. He has an exceptionally clear understanding of coding and problem solving. In the Robotics class, he chose to work with MicroPython in the Mindstorms setting. This was taking a definite risk and will prompt a curricular change to that language! Liz Gluz – Liz started off the term a bit slowly, but quickly made the changes in study habits necessary for success. She saw me frequently for extra help; she did all her homework problems and then even more for additional practice; and she was always one of the first students to raise her hand with questions during class. Her extra effort made all the difference, and her final test score of the fall term was one of the best in the whole class.
Liz Gluz – Liz started off the term a bit slowly, but quickly made the changes in study habits necessary for success. She saw me frequently for extra help; she did all her homework problems and then even more for additional practice; and she was always one of the first students to raise her hand with questions during class. Her extra effort made all the difference, and her final test score of the fall term was one of the best in the whole class. Anita Hua – Anita Hua is an exceptionally strong math student, but what makes her truly stand out is her genuine joyous passion for mathematics. She leverages her enthusiasm and academic gifts to conquer even the most complex material, and also help her peers reach the same level of understanding. She was actively engaged in class and on the math team even when she was connecting to classes remotely, and her success and large impact has continued as she transitioned to attending class in person. Bravo Anita!
Anita Hua – Anita Hua is an exceptionally strong math student, but what makes her truly stand out is her genuine joyous passion for mathematics. She leverages her enthusiasm and academic gifts to conquer even the most complex material, and also help her peers reach the same level of understanding. She was actively engaged in class and on the math team even when she was connecting to classes remotely, and her success and large impact has continued as she transitioned to attending class in person. Bravo Anita! Felix King – Felix has been a consistently strong AP Calculus student. He comes to class well prepared and ready to work. In class, he is always well focused and engaged in our discussions. He is always willing to lend his insights into the material with his classmates which helps the class move forward. His work ethic and devotion has been exemplary throughout the year.
Felix King – Felix has been a consistently strong AP Calculus student. He comes to class well prepared and ready to work. In class, he is always well focused and engaged in our discussions. He is always willing to lend his insights into the material with his classmates which helps the class move forward. His work ethic and devotion has been exemplary throughout the year. Andie Kinstle – Andie has been awesome overall in Honors Geometry. Her diagrams and explanations are clear, insightful, and easy for the entire group to follow. She is always willing to share her ideas and help others. She works hard not only to understand the concepts and problems, but also to clearly show this understanding.
Andie Kinstle – Andie has been awesome overall in Honors Geometry. Her diagrams and explanations are clear, insightful, and easy for the entire group to follow. She is always willing to share her ideas and help others. She works hard not only to understand the concepts and problems, but also to clearly show this understanding.  Calvin Klumpp – Calvin has been a leader during discussions. Calvin articulates his thoughts well, he challenges our thinking on concepts, and he gives us alternate ways of approaching problems. Calvin has provided the class with thought provoking ideas without dominating discussion. Not only has he contributed to discussions, but he also just looks out for the class. He will let the teacher know if the screen is still frozen, he will remind us to mute or unmute on Zoom, and he will help solve technical difficulties. His community approach to learner is refreshing and necessary!
Calvin Klumpp – Calvin has been a leader during discussions. Calvin articulates his thoughts well, he challenges our thinking on concepts, and he gives us alternate ways of approaching problems. Calvin has provided the class with thought provoking ideas without dominating discussion. Not only has he contributed to discussions, but he also just looks out for the class. He will let the teacher know if the screen is still frozen, he will remind us to mute or unmute on Zoom, and he will help solve technical difficulties. His community approach to learner is refreshing and necessary! Leila Minkara – This fall, despite being virtual, Leila was able to consistently demonstrate her understanding of trigonometry scoring near the top of her class on most assessments. Additionally, Leila did all her work with great detail and always managed to turn it in even when surface issues occurred. Due to her resilience, hard work, and positive attitude I am proud to nominate Leila as my math student of the trimester.
Leila Minkara – This fall, despite being virtual, Leila was able to consistently demonstrate her understanding of trigonometry scoring near the top of her class on most assessments. Additionally, Leila did all her work with great detail and always managed to turn it in even when surface issues occurred. Due to her resilience, hard work, and positive attitude I am proud to nominate Leila as my math student of the trimester.  Sean O’Donnell – Sean has completed an exceptional trimester in Geometry. He is intellectually curious, a diligent worker and a leader with his outstanding class participation. He has strong natural instincts for geometry and his deductive reasoning skills are excellent. Always ready to explain how an answer can be found, Sean supports each of his statements with sound mathematical reasoning and enthusiasm. Congratulations, Sean, on this well earned award!
Sean O’Donnell – Sean has completed an exceptional trimester in Geometry. He is intellectually curious, a diligent worker and a leader with his outstanding class participation. He has strong natural instincts for geometry and his deductive reasoning skills are excellent. Always ready to explain how an answer can be found, Sean supports each of his statements with sound mathematical reasoning and enthusiasm. Congratulations, Sean, on this well earned award!  Lorenzo Redaelli – Lorenzo has done a wonderful job this fall – adjusting to class in the gym, zoom, and a new school. He has excelled academically and has worked to help others in class. He asks great questions and contributes to the class conversation. He is a leader in class.
Lorenzo Redaelli – Lorenzo has done a wonderful job this fall – adjusting to class in the gym, zoom, and a new school. He has excelled academically and has worked to help others in class. He asks great questions and contributes to the class conversation. He is a leader in class.  Nick Sobon – Nick demonstrated a fantastic work ethic during the first trimester. In class, he was always engaged and was not shy about volunteering and asking clarifying questions. Additionally, Nick set up extra help sessions on numerous occasions and developed a plan for what was going to make him successful in Geometry. The work that he handed in was also a reflection of his commitment to trying his best. Nick sets a positive example for others and is a pleasure to have in class!
Nick Sobon – Nick demonstrated a fantastic work ethic during the first trimester. In class, he was always engaged and was not shy about volunteering and asking clarifying questions. Additionally, Nick set up extra help sessions on numerous occasions and developed a plan for what was going to make him successful in Geometry. The work that he handed in was also a reflection of his commitment to trying his best. Nick sets a positive example for others and is a pleasure to have in class!  Sydney Wegner – Sydney showed determination to not only do well, but to truly comprehend all the material. She came to every class attentive and engaged. Sydney showed her peers that giving up on a problem is not an option.
Sydney Wegner – Sydney showed determination to not only do well, but to truly comprehend all the material. She came to every class attentive and engaged. Sydney showed her peers that giving up on a problem is not an option.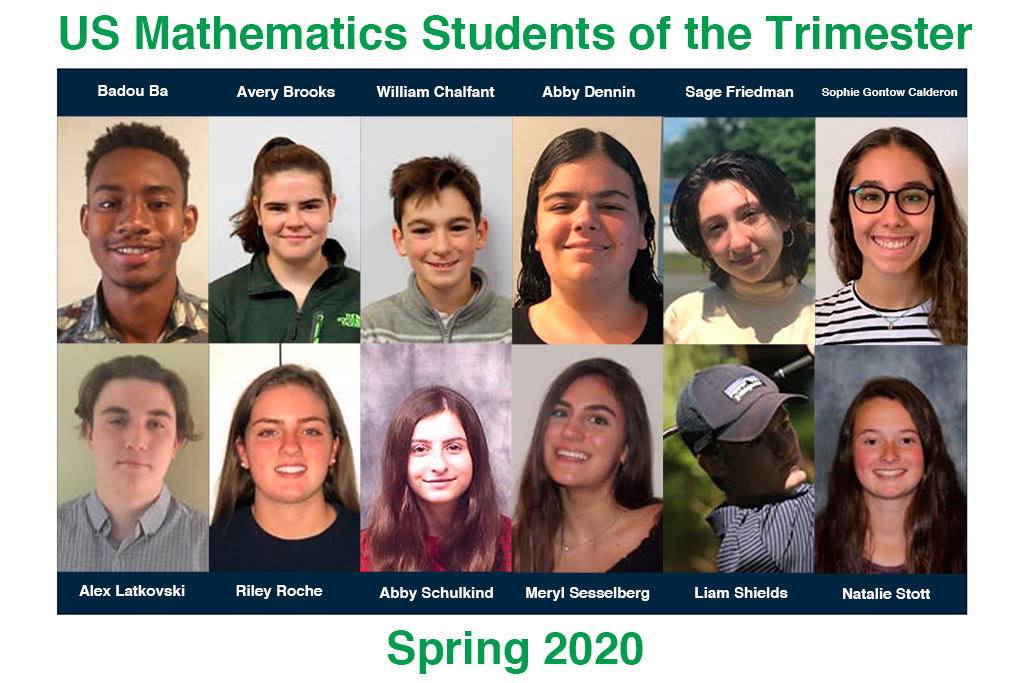
 Badou Ba – Badou’s commitment to developing as a programmer came to a fruition this trimester. His work in coding in a new language (his third of this year!) showed considerable growth and understanding. He showed both independence and perseverance as he worked through a wide variety of challenging problems.
Badou Ba – Badou’s commitment to developing as a programmer came to a fruition this trimester. His work in coding in a new language (his third of this year!) showed considerable growth and understanding. He showed both independence and perseverance as he worked through a wide variety of challenging problems. Avery Brooks – Avery has just completed one amazing trimester as a student of geometry under the most unique circumstances. As we entered the unknown and sometimes unpredictable world of remote learning, Avery was a role model for her classmates by coming out of her comfort zone and volunteering multiple times each class. Avery’s determination to be her best was evident daily and her consistent effort in and out of class led to her best quiz results of the year. Always engaged, Avery set a positive tone in class and thrived on Zoom. Thank you, Avery, for your leadership and congratulations on this well deserved honor!
Avery Brooks – Avery has just completed one amazing trimester as a student of geometry under the most unique circumstances. As we entered the unknown and sometimes unpredictable world of remote learning, Avery was a role model for her classmates by coming out of her comfort zone and volunteering multiple times each class. Avery’s determination to be her best was evident daily and her consistent effort in and out of class led to her best quiz results of the year. Always engaged, Avery set a positive tone in class and thrived on Zoom. Thank you, Avery, for your leadership and congratulations on this well deserved honor! William Chalfant – Will struggled with math for the first two trimesters, but thrived in T3. He attended help sessions, lead his peers, and demonstrated a deep understanding of the material. He mentioned that this was his best trimester in math to date and that he is incredibly proud of himself. Will is a hard worker and is incredibly motivated, not to mention kind and fun to have in class.
William Chalfant – Will struggled with math for the first two trimesters, but thrived in T3. He attended help sessions, lead his peers, and demonstrated a deep understanding of the material. He mentioned that this was his best trimester in math to date and that he is incredibly proud of himself. Will is a hard worker and is incredibly motivated, not to mention kind and fun to have in class. Abigail Dennin – Abby stepped up on multiple fronts to help drive class during a new and challenge time! She helped create new content, manage groups of students, and go above and beyond during class discussions. She was committed, and poured genuine interest into new types of assignments, thriving independently as well as in teams. He passion for mathematics and rising to the challenge of the times was impressive and noted by everyone in class!
Abigail Dennin – Abby stepped up on multiple fronts to help drive class during a new and challenge time! She helped create new content, manage groups of students, and go above and beyond during class discussions. She was committed, and poured genuine interest into new types of assignments, thriving independently as well as in teams. He passion for mathematics and rising to the challenge of the times was impressive and noted by everyone in class! Sage Friedman – Sage has consistently been an excellent contributor in and out of class. Her positive spirit and determination did not fade at all, even with the transition to online learning. She continued asking insightful questions, completed her homework with the same degree of thoroughness, and demonstrated exceptional understanding of concepts. Congratulations Sage!
Sage Friedman – Sage has consistently been an excellent contributor in and out of class. Her positive spirit and determination did not fade at all, even with the transition to online learning. She continued asking insightful questions, completed her homework with the same degree of thoroughness, and demonstrated exceptional understanding of concepts. Congratulations Sage! Sophie Gontow-Calderon – Sophie had a fabulous aspring term in Honors Discrete. Her understanding of the graph theory we studied during the spring term was truly remarkable. Moreover, she always supplements her fantastic mathematical insight with exceptional diligence and effort.
Sophie Gontow-Calderon – Sophie had a fabulous aspring term in Honors Discrete. Her understanding of the graph theory we studied during the spring term was truly remarkable. Moreover, she always supplements her fantastic mathematical insight with exceptional diligence and effort. Alex Latkovski – Alex did a remarkable job this year, becoming a serious student. While we learned through zoom this spring, Alex made extra effort to ask questions and participate from a distance. It was a pleasure to work with him throughout the year as he developed and took ownership of his education.
Alex Latkovski – Alex did a remarkable job this year, becoming a serious student. While we learned through zoom this spring, Alex made extra effort to ask questions and participate from a distance. It was a pleasure to work with him throughout the year as he developed and took ownership of his education. Riley Roche – Riley showed the highest level of commitment to learn new material throughout the trimester. Her determination to challenge herself was unmatched. She never let down. She has my highest praise for not only pressing on during a trying time but succeeding at the highest level.
Riley Roche – Riley showed the highest level of commitment to learn new material throughout the trimester. Her determination to challenge herself was unmatched. She never let down. She has my highest praise for not only pressing on during a trying time but succeeding at the highest level. Abby Schulkind – Abby has been a consistently diligent and hardworking math student all year long. Abby has shown genuine curiosity and enthusiasm for AP Calculus. She always wants to learn more and to fully understand the concepts that we study.
Abby Schulkind – Abby has been a consistently diligent and hardworking math student all year long. Abby has shown genuine curiosity and enthusiasm for AP Calculus. She always wants to learn more and to fully understand the concepts that we study. Meryl Sesselberg – Meryl continued her excellent work in Honors Geometry during T3. She is a great, independent worker who was unfazed by online learning. She referred back to previous learning to advance her own knowledge, making great connections and showing detailed explanations.
Meryl Sesselberg – Meryl continued her excellent work in Honors Geometry during T3. She is a great, independent worker who was unfazed by online learning. She referred back to previous learning to advance her own knowledge, making great connections and showing detailed explanations. Liam Shields – During T3 of AP CSP, Liam continued to bring his energy, interest, and enthusiasm to our virtual classes every meeting. He also poured himself into his final coding project and recreated part of one of his favorite classic games: The Legend of Zelda!
Liam Shields – During T3 of AP CSP, Liam continued to bring his energy, interest, and enthusiasm to our virtual classes every meeting. He also poured himself into his final coding project and recreated part of one of his favorite classic games: The Legend of Zelda! Natalie Stott – Despite the challenges faced this trimester, Natalie thrived putting together her most successful trimester this year. Natalie attended every required class, asked questions, and had strong performances on each of the assessments. For these reasons and more I am more then happy to make Natalie my math student of the trimester.
Natalie Stott – Despite the challenges faced this trimester, Natalie thrived putting together her most successful trimester this year. Natalie attended every required class, asked questions, and had strong performances on each of the assessments. For these reasons and more I am more then happy to make Natalie my math student of the trimester.




 Brie Banas – Brie has been a consistently strong math student throughout the year. She is intelligent, hard working and diligent yet very unassuming. She is always willing to lend her thoughtful insights into the material with her fellow classmates which consistently helps propel our class forward.
Brie Banas – Brie has been a consistently strong math student throughout the year. She is intelligent, hard working and diligent yet very unassuming. She is always willing to lend her thoughtful insights into the material with her fellow classmates which consistently helps propel our class forward. Grace Bean – Grace has had an amazing second trimester. Always a class leader in terms of participation, Grace has stretched herself on the most difficult “”ticket to the weekend “” problems. On more than one occasion, she has been the first student to complete these challenge problems. She is quick to infer previously learned concepts into new problems and she shares her thoughts enthusiastically with the classmates. Grace is always ready to learn and grow.
Grace Bean – Grace has had an amazing second trimester. Always a class leader in terms of participation, Grace has stretched herself on the most difficult “”ticket to the weekend “” problems. On more than one occasion, she has been the first student to complete these challenge problems. She is quick to infer previously learned concepts into new problems and she shares her thoughts enthusiastically with the classmates. Grace is always ready to learn and grow. Anfisa Bogdanenko – In our final section on recursion, Anfisa work to create a recursive backtracking algorithm to solve Slitherlink puzzles. She took this challenge precisely because it was not readily apparent how it would work. Throughout, her analysis of the problem was incredibly insightful and ultimately led to a working solution! Very daring and very bold!
Anfisa Bogdanenko – In our final section on recursion, Anfisa work to create a recursive backtracking algorithm to solve Slitherlink puzzles. She took this challenge precisely because it was not readily apparent how it would work. Throughout, her analysis of the problem was incredibly insightful and ultimately led to a working solution! Very daring and very bold! Elsa Frankel – Elsa has led the class by entering into many discussions about challenging problems. Her classmates appreciate looking at her work because it is easy to read and clear when following her steps. Finally, when I had to miss a class, I reached out to Elsa to make sure class got started properly. She went beyond expectations by providing a summary of the class discussion- what went well and we should still go over more. She’s thorough and precise in all that she does! She is always willing to help.
Elsa Frankel – Elsa has led the class by entering into many discussions about challenging problems. Her classmates appreciate looking at her work because it is easy to read and clear when following her steps. Finally, when I had to miss a class, I reached out to Elsa to make sure class got started properly. She went beyond expectations by providing a summary of the class discussion- what went well and we should still go over more. She’s thorough and precise in all that she does! She is always willing to help.  Liz Gluz – Liz has worked hard all year in and out of the classroom. She has gone above trying to just understand the basics. She excelled at seeing all the connections in each lesson and has shown a high level of mastery.
Liz Gluz – Liz has worked hard all year in and out of the classroom. She has gone above trying to just understand the basics. She excelled at seeing all the connections in each lesson and has shown a high level of mastery. Lauren Goldinger – Academically, Lauren was consistently at or near the top of her class this winter. This success was due to consistent hard work and perseverance when faced with new mathematical challenges. Additionally, Lauren regularly made a positive impact in our classroom though her ability to collaborate productively with anyone during group work sessions. For the reasons above and more I am proud to make Lauren my math student of the trimester.
Lauren Goldinger – Academically, Lauren was consistently at or near the top of her class this winter. This success was due to consistent hard work and perseverance when faced with new mathematical challenges. Additionally, Lauren regularly made a positive impact in our classroom though her ability to collaborate productively with anyone during group work sessions. For the reasons above and more I am proud to make Lauren my math student of the trimester. Adam Johnsrud – Adam is a proactive learner. He goes out of his way to be prepared and lean into challenging material. He is actively kind, naturally makes space for others, and he goes out of his way to ask people how they are doing, and really care about their response. Adam is a model math student and a fantastic all-around human.
Adam Johnsrud – Adam is a proactive learner. He goes out of his way to be prepared and lean into challenging material. He is actively kind, naturally makes space for others, and he goes out of his way to ask people how they are doing, and really care about their response. Adam is a model math student and a fantastic all-around human. Sarah Kimmel – Sarah is a stellar computer scientist! She brings a natural curiosity and joy for learning to class. She’s a positive contributor to the class discourse and challenges herself during homework and project assignments.
Sarah Kimmel – Sarah is a stellar computer scientist! She brings a natural curiosity and joy for learning to class. She’s a positive contributor to the class discourse and challenges herself during homework and project assignments. Kam LaFountain – Kam has worked so diligently this year in Geometry Essentials. He has been prepared every day, and he seeks me out for help as soon as any information is unclear. He’s always totally on task during class time, and he obviously really wants to learn all the material.
Kam LaFountain – Kam has worked so diligently this year in Geometry Essentials. He has been prepared every day, and he seeks me out for help as soon as any information is unclear. He’s always totally on task during class time, and he obviously really wants to learn all the material. Matt Pimental – Matt has been a great student throughout the year. He is a leader during discussions, even taking over class one day when my throat limited my speaking. He does great work on projects and is always putting forth his best effort.
Matt Pimental – Matt has been a great student throughout the year. He is a leader during discussions, even taking over class one day when my throat limited my speaking. He does great work on projects and is always putting forth his best effort. Hannah Roche – Hannah puts a lot of thought and care into her work both in and out of class. She is always prepared and sets a great example for her classmates.
Hannah Roche – Hannah puts a lot of thought and care into her work both in and out of class. She is always prepared and sets a great example for her classmates. Tyler Varin – Tyler did a particularly nice job during the second trimester, stepping up as a leader in this class. Tyler was always ready to participate in class discussions and was willing to take risks with challenging material. His trimester minimization project was outstanding.
Tyler Varin – Tyler did a particularly nice job during the second trimester, stepping up as a leader in this class. Tyler was always ready to participate in class discussions and was willing to take risks with challenging material. His trimester minimization project was outstanding.