Origami is one of the primary reasons I have devoted my life to mathematics. When I was 5, my parents gave me my first Origami books and I was immediately hooked. I can remember waking up early every morning to fold all sorts of models. The geometry, logic, necessary dexterity, and focus were all mesmerizing.
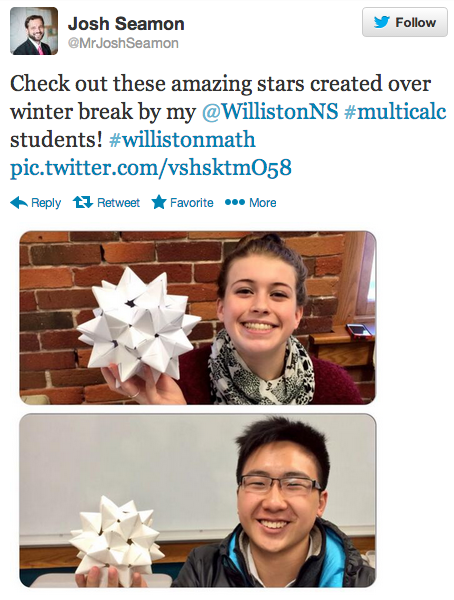
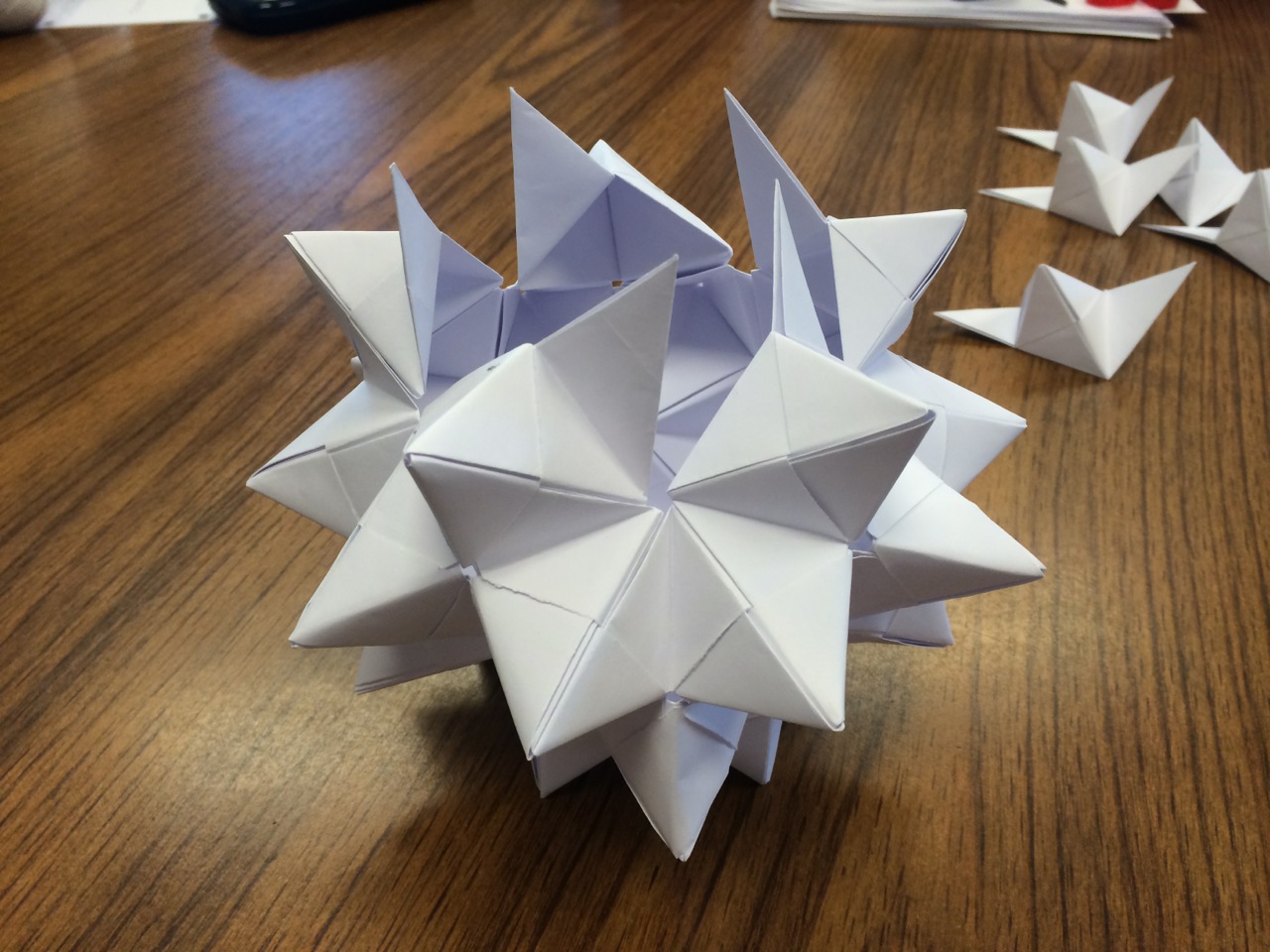
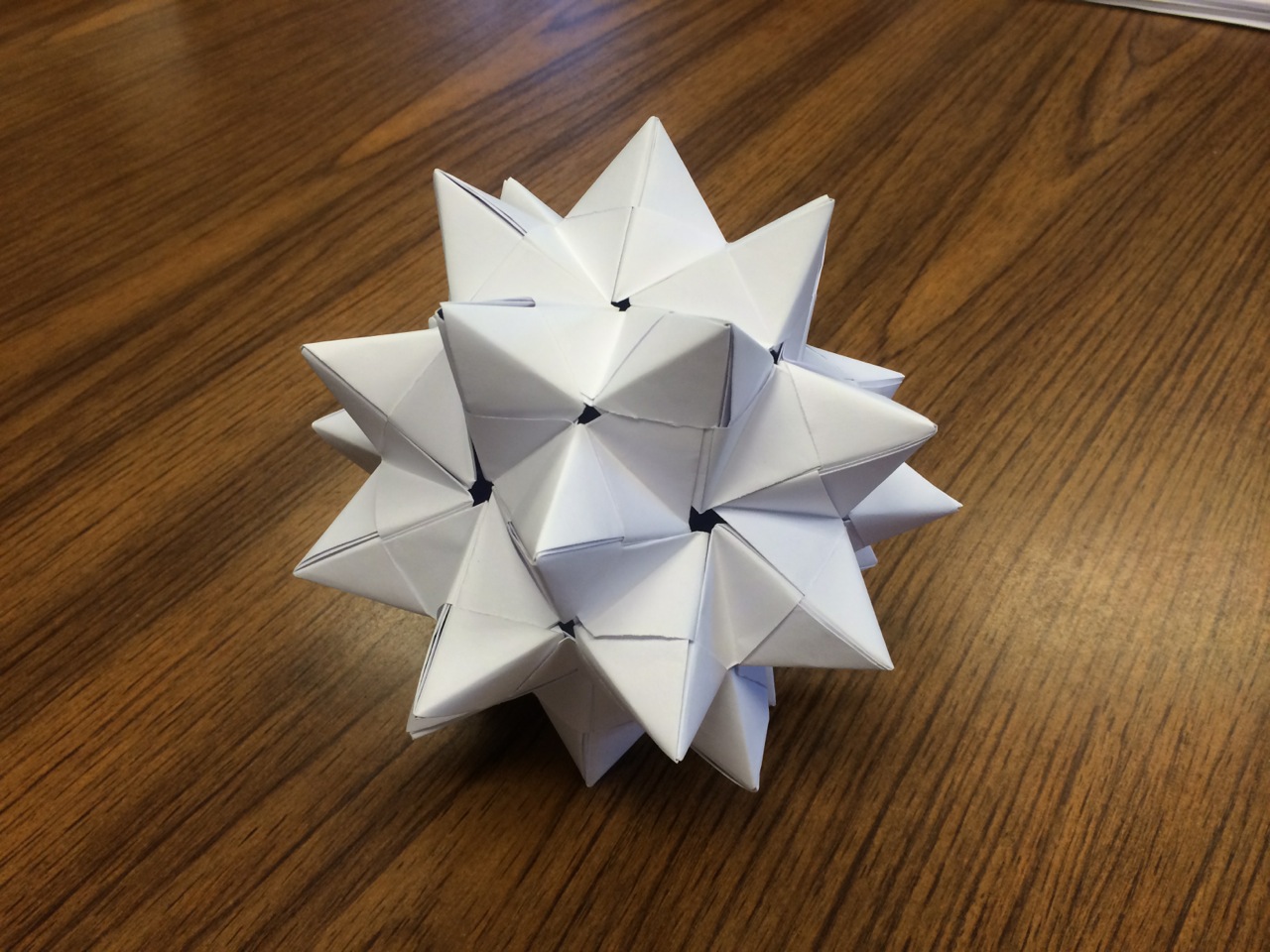
Origami has been a constant in just about all of my math courses. We have constructed specific angles in Geometry, studied complex surface area and volume questions with the help of modular Origami in Multivariable Calculus, and worked on edge connectivity problems in Algebra 2. There are seemingly infinite numbers of ways to work Origami into just about any math lesson! You can read about a recent class module right here: Star Project Part 1, Part 2, Part 3, and Part 4.

Not only can Origami be applied almost anywhere in math, it’s incredibly fun. We’re only a few weeks into class and I’ve already had several students approach me to ask about when we’ll be folding. I can’t wait to get started with this year’s students!
Last fall, as part of my quest to learn as much as I can about Origami, I sat down with one of the world leaders of Math and Origami, Dr. Thomas Hull, Associate Professor at Western New England University and author of Project Origami: Activities for Exploring Mathematics. Dr. Hull was, as expected, provided an absolute wealth of information! We spoke about how to best design Origami lessons, how to choose topics, as well as his recent sabbatical trip to Japan. His input was invaluable in helping me design my Williston professional development application to travel to Japan and study Origami!
 Williston accepted my proposal and I was able to travel to Japan in August to attend the Origami Tanteidan in Tokyo. Needless to say, I had an incredible time!
Williston accepted my proposal and I was able to travel to Japan in August to attend the Origami Tanteidan in Tokyo. Needless to say, I had an incredible time!